Annals of Civil and Environmental Engineering

ISSN: 2574-0350

Research Article

Study the effect of transient vibration on multi-storey building structure according to equivalent spring-mass system performed by Ansys

Dinesh Sen¹ and Anosh Kujur^2*

¹Assistent Professor and HOD, Civil Engineering, VEC Lakhanpur, Chhattisgarh, India
²M-Tech Student, Department of Civil Engineering, VEC Lakhanpur, Chhattisgarh, India

*Address for Correspondence: Anosh Kujur, M-Tech Student, Department of Civil Engineering, VEC Lakhanpur, Chhattisgarh, India, Email: anosh.kujur@gmail.com

Dates: Submitted: 04 January 2019; Approved: 15 April 2019; Published: 16 April 2019

How to cite this article: Sen D, Kujur A. Study the effect of transient vibration on multi-storey building structure according to equivalent spring-mass system performed by Ansys. Ann Civil Environ Eng. 2019; 3: 011-024. DOI: 10.29328/journal.acee.1001013

Copyright License: © 2019 Sen D, et al. This is an open access article distributed under the Creative Commons Attribution License, which permits unrestricted use, distribution, and reproduction in any medium, provided the original work is properly cited.

Keywords: Transient vibration response; MDOF; Time-history graph; Excitation; Spring-mass system; Undamped of forced system; Damped of forced system; Underdamped system; Critically damped system; Overdamped system; Natural frequency; Modelling and Simulation; ANSYS; Mode superposition method

Vibration is a physical phenomenon of any structure. In the case of forced vibrations, the structure is made to vibrate under the control of external force. Commonly in engineering practice, there are three types of forcing functions, i.e. periodic, impulsive and random forcing functions. Impulsive forcing function cause transient vibration. Transient response analysis is the method for computing forced dynamic response. The purpose of a transient response analysis is to compute the behaviour of a structure subjected to time-varying excitation. The transient vibration makes the highly sudden impact which causes our structures is the collapse. The transient response analysis provides sufficient damping result for structures which resist various human-made or natural forces like earthquakes, tsunami, and blast and machine vibrations.

A multi-degree of freedom system (MDOF) may be a multi-storey building, a TV tower, a Bridge including its super-structure and sub-structure, a flexural member, a machine foundation, an underground metro station etc.

In this study according to the equivalent mass-spring system of the 4-storey building has been studied the transient vibration response. ANSYS software is used to perform the analysis. Before start the transient analysis, natural frequency has been known by ANSYS, and it has been compared with theoretical results to check the validation of the software.

Determination of natural frequency

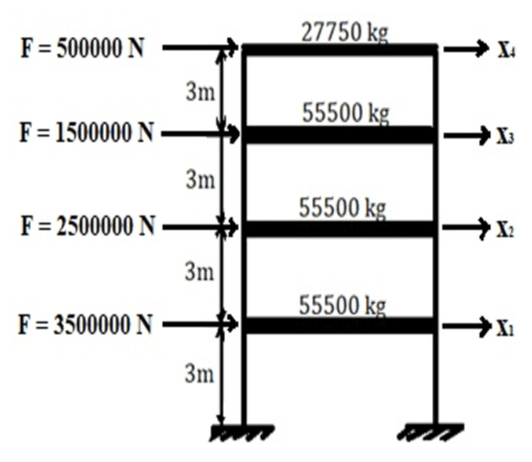
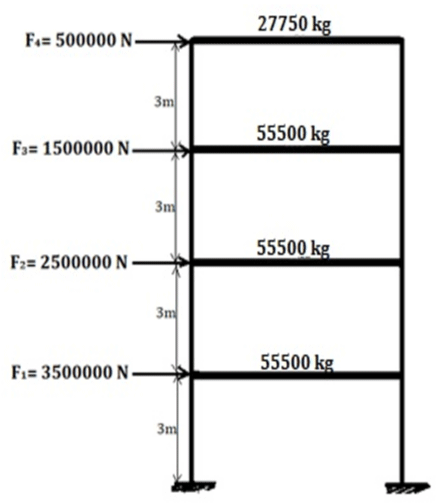
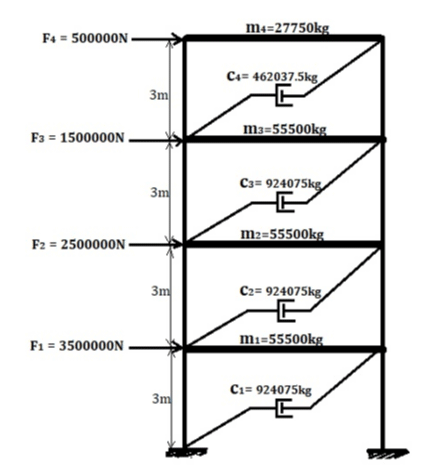
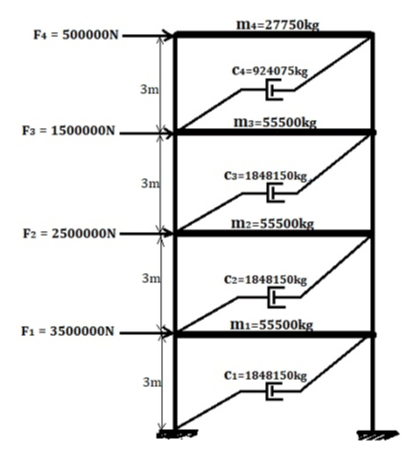
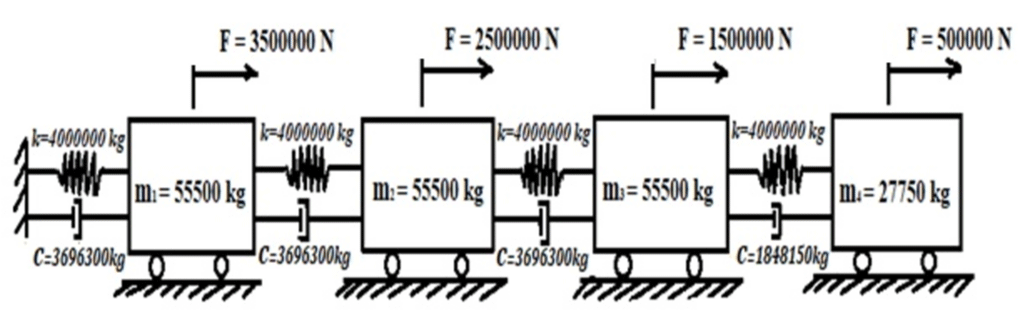
A four storey building masses of floor m₁ =m₂ = m₃ = 55500 kg and m₄ = 27750 kg building height of each floor is 3m, Forced applied in the 1^st, 2^nd, 3^rd, storey i.e. F₁ = 3500000 N, F₂ = 2500000 N, F₃ = 1500000 N and F₄ = 500000 N, stiffness provided in each storey is 4x 10⁶N/m (Figure 1).

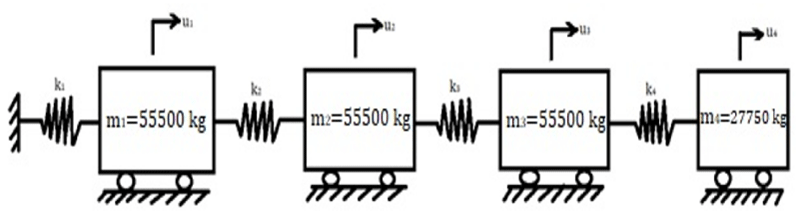
Determine Natural Frequencies by the Matrix Method (Figures 2.1 - 2.6):

Masses is given as m₁ = 55500 kg, m₂ = 55500 kg, m₃ = 55500 kg, m₄ = 27750 kg

${\text{K}}_{\text{1}}={\text{ k}}_{\text{2}}={\text{ k}}_{\text{3}}={\text{ k}}_{\text{4}}=\text{ 2}\times \frac{12EI}{L}=\frac{2\times 12\times 4.5\times {10}^6}{3^3}=4\times {10}^6\text{ N}/\text{m}$

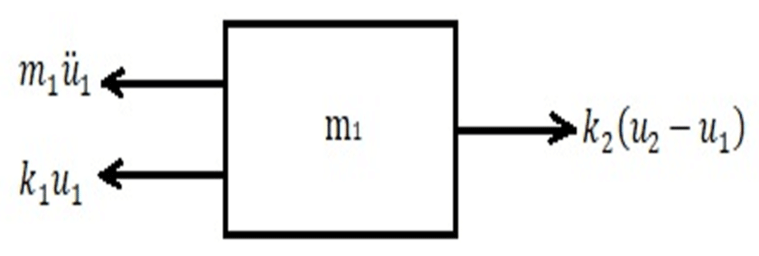
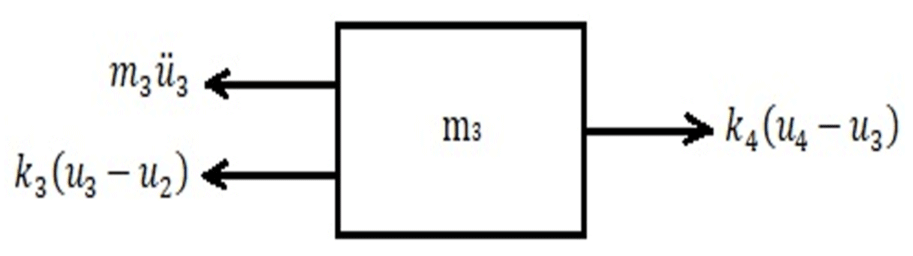
The following equation obtained from free body diagram

$m_1{\ddot{u}}_1+\left(k_1+k_2\right)u_1- k_2{u}_2=0\text{ (1)}$

$m_2{\ddot{u}}_2-k_2{u}_1+\left(k_2+k_3\right)u_2-k_3{u}_3=0\text{ (2)}$

$m_3{\ddot{u}}_3-k_3{u}_2+\left(k_3+k_4\right)u_3-k_4{u}_4=0\text{ (3)}$

$m_4{\ddot{u}}_4-k_4{u}_3+k_4{u}_4=0\text{ (4)}$

Write the equations (1), (2), (3) & (4) into the equation of motion of an MDOF system subjected to undamped free vibration is given as

$\left[m\right]\left\{\ddot{u}\right\}+\left[k\right]\left\{u\right\}= \left\{0\right\}\text{ (5)}$

$\left[\begin{array}{c}\begin{array}{cc}{m}_1& \begin{array}{cc}0& \begin{array}{cc}0& 0\end{array}\end{array}\end{array}\\ \begin{array}{cc}0& \begin{array}{cc}{m}_2& \begin{array}{cc}0& 0\end{array}\end{array}\end{array}\\ \begin{array}{cc}\begin{array}{c}0\\ 0\end{array}& \begin{array}{c}\begin{array}{cc}0& \begin{array}{cc}{m}_3& 0\end{array}\end{array}\\ \begin{array}{cc}0& \begin{array}{cc}0& m_4\end{array}\end{array}\end{array}\end{array}\end{array}\right]\left\{\begin{array}{c}{\ddot{u}}_1\\ {\ddot{u}}_2\\ \begin{array}{c}{\ddot{u}}_3\\ {\ddot{u}}_4\end{array}\end{array}\right\}+\left[\begin{array}{cc}\begin{array}{cc}\left(k_1+k_2\right) & -k_2\end{array}& \begin{array}{cc}0& 0\end{array}\\ \begin{array}{cc}-k_2& (k_2+k_3)\\ \begin{array}{c}0\\ 0\end{array}& \begin{array}{c}-k_3\\ 0\end{array}\end{array}& \begin{array}{c}\begin{array}{cc}-k_3& 0\end{array}\\ \begin{array}{c}\begin{array}{cc}(k_3+k_4)& -k_4\end{array}\\ \begin{array}{cc}-k_4& k_4\end{array}\end{array}\end{array}\end{array}\right]\left\{\begin{array}{c}\begin{array}{c}{u}_1\\ u_2\end{array}\\ u_3\\ u_4\end{array}\right\}=0\text{ (6)}$

$\text{1}000\left[\begin{array}{cccc}55.5& 0& 0& 0\\ 0& 55.5& 0& 0\\ 0& 0& 55.5& 0\\ 0& 0& 0& 27.75\end{array}\right]\left\{\begin{array}{c}{\ddot{u}}_1\\ {\ddot{u}}_2\\ \begin{array}{c}{\ddot{u}}_3\\ {\ddot{u}}_4\end{array}\end{array}\right\}+4\times {10}^6\left[\begin{array}{cccc}2& -1& 0& 0\\ -1& 2& -1& 0\\ 0& -1& 2& -1\\ 0& 0& -1& 1\end{array}\right]\left\{\begin{array}{c}{u}_1\\ u_2\\ \begin{array}{c}{u}_3\\ u_3\end{array}\end{array}\right\}=0\text{ (7)}$

The characteristic equation is $\left|\left(k\right)-(m){\omega }_n^2\right|=0$

$\left|4\times {10}^2\left[\begin{array}{l}2\text{ -1 0 0}\\ \text{-1 2 -1 0}\\ \text{0 -1 2 -1}\\ \text{0 0 -1 1}\end{array}\right]-1000{\omega }_n^2\left[\begin{array}{l}55.5\text{ 0 0 0}\\ \text{0 55}\text{.5 0 0}\\ \text{0 0 55}\text{.5 0}\\ 0\text{ 0 0 27}\text{.75}\end{array}\right]\right|=0\text{ (8)}$

$\left|\left[\begin{array}{l}2\text{ -1 0 0}\\ \text{-1 2 -1 0}\\ \text{0 -1 2 -1}\\ \text{0 0 -1 1}\end{array}\right]--2.5\times {10}^{-4}{\omega }_n^2\left[\begin{array}{l}55.5\text{ 0 0 0}\\ \text{0 55}\text{.5 0 0}\\ \text{0 0 55}\text{.5 0}\\ 0\text{ 0 0 27}\text{.75}\end{array}\right]\right|=0\text{ (9)}$

Let 2.5×$\times {10}^{-4}{\omega }_n^2=\lambda$ we get

$\left|\left[\begin{array}{ccc}(2-55.5\lambda )& -1& \begin{array}{cc}0& 0\end{array}\\ -1& (2-55.5\lambda )& \begin{array}{cc}-1& 0\end{array}\\ \begin{array}{c}0\\ 0\end{array}& \begin{array}{c}-1\\ 0\end{array}& \begin{array}{c}\begin{array}{cc}(2-55.5\lambda )& -1\end{array}\\ \begin{array}{cc}-1& (1-27.75\lambda )\end{array}\end{array}\end{array}\right]\right|=0\text{ (10)}$

Expanding the determinant, we get

4743970.031λ4 – 683815.5λ3 + 30802.5λ2 - 444λ + 1 = 0 [11)

Solving the above equation, we get

λ¹ = 0.00274308 = λ² = 0.0222456 = λ³ = 0.0498264 = λ⁴ = 0.069329

We know that 2.5$\times {10}^{-4}{\omega }_n^2=\lambda$

Thus 2.5$\times {10}^{-4}{\omega }_1^2={\lambda }_1=0.00274308$

${\omega }_1^2= \frac{0.00274308}{2.5\times {10}^{-4}}$ = ${\omega }_1=3.3125\frac{rad}{s}=0.53 Hz$

${\omega }_2^2= \frac{0.0222456}{2.5\times {10}^{-4}}\text{ =}$ ${\omega }_2=9.433\frac{rad}{s}=1.5 Hz$

${\omega }_3^2= \frac{0.0498264}{2.5\times {10}^{-4}}\text{ =}$ ${\omega }_3=14.12\frac{rad}{s}=2.25 Hz$

${\omega }_4^2= \frac{0.069329}{2.5\times {10}^{-4}}=$ ${\omega }_4=16.6528\frac{rad}{s}=2.65 Hz$

The Natural frequencies (or) Eigen values are

${\omega }_1=0.53 Hz$

${\omega }_2=1.5 Hz$

${\omega }_3=2.25 Hz$

${\omega }_4=2.65 Hz$

Determination of natural frequencies by ANSYS

Material and Geometry: Structural steel used for making masses m₁, m₂, m₃ and m₄ with density 7850 kg/m^3 and young modulus and Poisson’s ratio of steel is 2×〖10〗^11Pa and 0.3 after that draw geometry by open Model dialog box from analysis systems of the toolbox and attach springs and provide stiffness value to all as 4×〖10〗^6N/m.

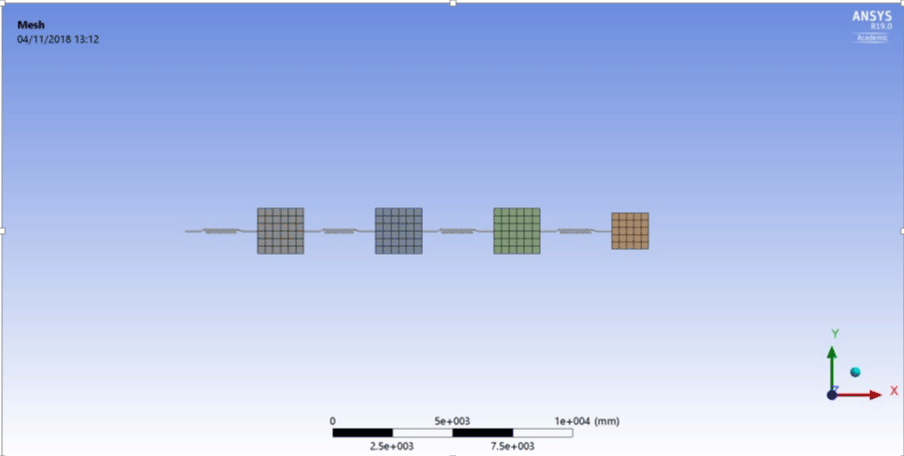
Meshing: Meshing divides the whole components into many small elements to distribute applied load uniformly to whole components. All faces were selected for mesh generation and the total number of nodes and elements were observed at 4431 and 773 respectively.

Frequencies obtained by ANSYS: The obtained frequency results are shown in table 1 Mode and Frequency.

Comparison of Theoretical calculation and ANSYS: The theoretical solved result almost matched with ANSYS results. Hence solution from theoretical solved results is valid and acceptable.

Transient vibration response of undamped of forced system using ansys

The 4-storey undamped of the forced system shown in figure 3.1

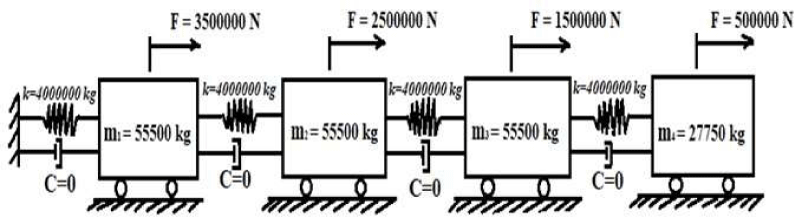
Equivalent undamped Spring-Mass system of 4-storey building with external applied force:

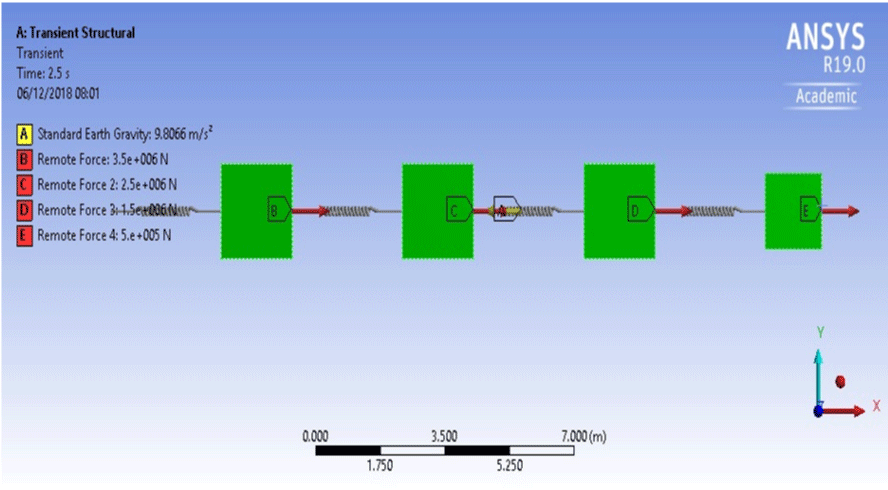
Equivalent Spring-mass model in ANSYS for 4-DOF Undamped of Forced System:

4-DOF Transient Vibration Response graph for Undamped of Forced System obtained by ANSYS:

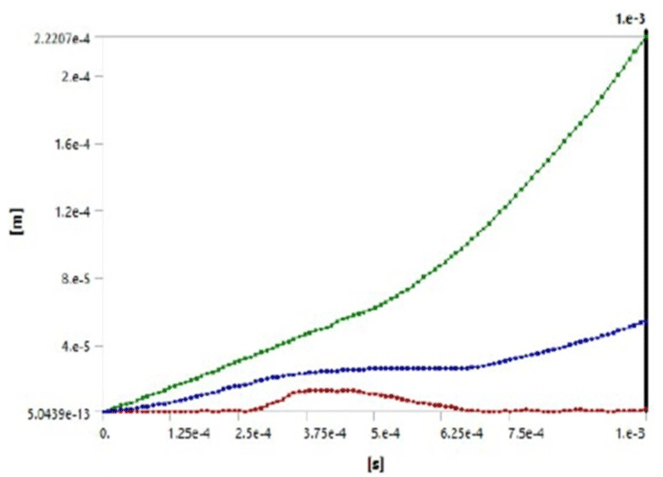
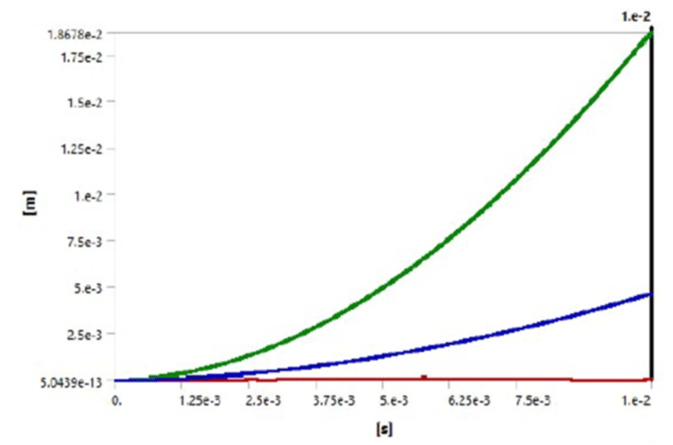
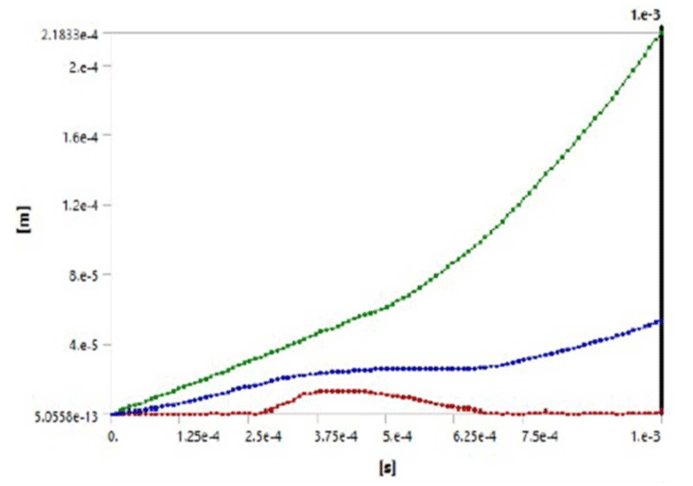
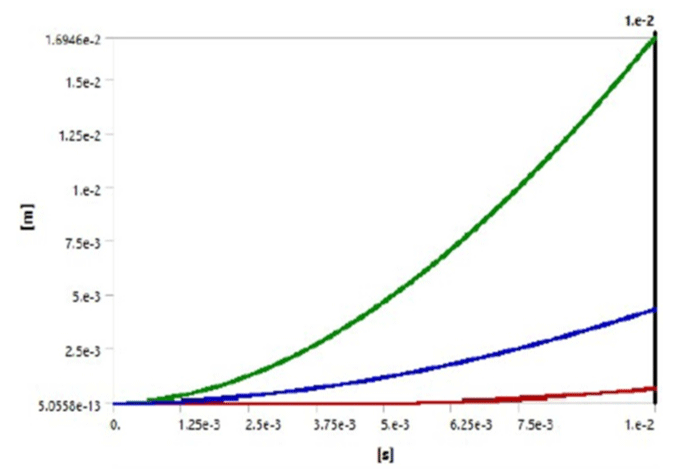
The 4-DOF Transient Vibration Response for (t) = 0.001 sec, (t) = 0.01 sec, initial time has taken 0.000001 sec.

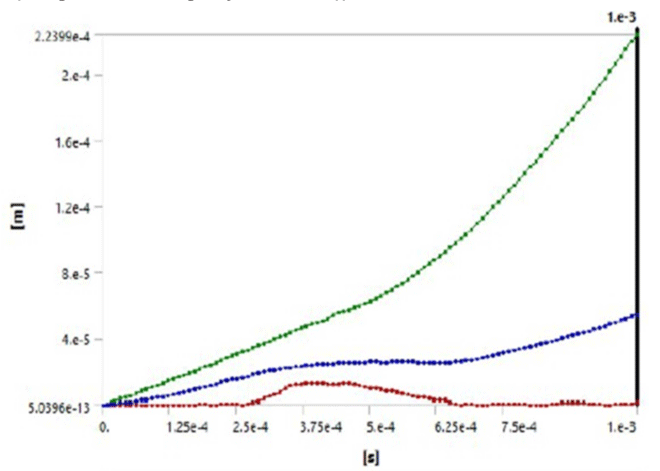
At the time (t) = 0.001 sec: The Transient Vibration Response for 4-DOF Undamped system obtained by ANSYS has shown below.

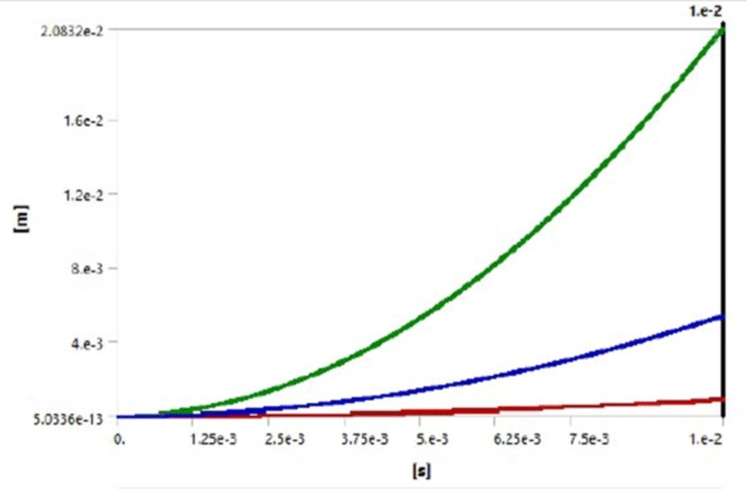
At time (t) =0.01 sec:

Analysis result for mdof forced vibration of undamped system

• Result for Max. & Min. Transient Response of MDOF forced vibration of Undamped system at two different time stages, i.e. (t)₁ = 0.001 sec, (t)₂ = 0.01 sec, presented in table 2.

• Maximum displacement over time (t) =0.01 sec presented in table 2.1

Transient vibration response for damped of forced system using ansys

4-dof transient vibration response for damped of forced system:

For Underdamped system:

If the damping ratio (ρ) = $\frac{c}{2m{\omega }_n}$ < 1.0 it is called underdamped system.

Where c = damping & ωn = natural damping in rad/sec

Provide damping for maximum frequency i.e. ω_n = 2.65Hz =16.65 rad/sec.

Taking Damping value c₁ = c₂ = c₃ = 924075 N-s/m for 1^st, 2^nd, and 3^rd storey & c₄ =462037.5 N-s/m for 4^th storey.

Check 1^st, 2^nd, & 3^rd storey damping:

Damping ratio (ρ) = $\frac{c}{2m{\omega }_n}=\frac{924075}{2\times 55500\times 16.65}$ 0.5 < 1 Hence 1^st, 2^nd, & 3^rd storey is underdamped.

Check 3^rd storey damping:

Damping ratio (ρ) =$\frac{c}{2m{\omega }_n}=\frac{462037.5}{2\times 27750\times 16.65}=0.5$ < 1 Hence 3^rd storey is underdamped.

Equivalent Spring-mass system for underdamped of forced system: Equivalent spring-mass system for 4-storey building in the underdamped condition.

4-DOF Transient Vibration Response graph for underdamped of Forced System obtained by ANSYS: The 4-DOF Transient Vibration Response for (t) = 0.001 sec, (t) = 0.01 sec, initial time is 0.000001 sec.

Graph for underdamped system at time (t) = 0.001 sec

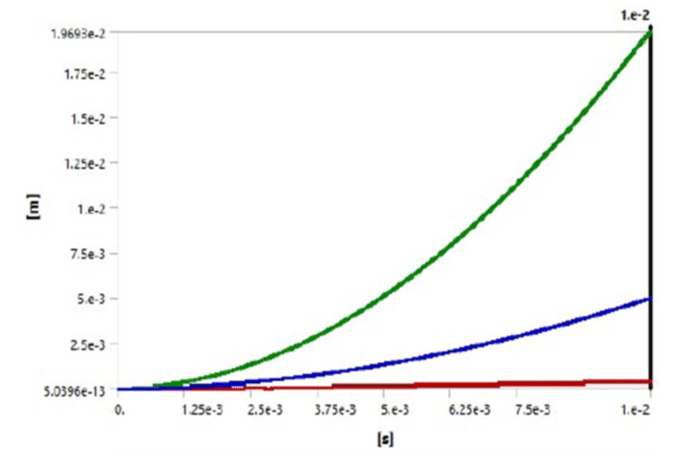
Graph for underdamped system at time (t) = 0.01 sec

Result for MDOF Underdamped Transient Response:

• Result for Max. & Min. Underdamped Transient Response of MDOF forced vibration of damped system at three different time stages, i.e. (t)₁ = 0.001sec, (t)₂ = 0.01 sec, presented in table 4.1

• Underdamped Maximum displacement over time (t) =0.01 sec presented in table 4.2

For critically damped of forced vibration system

Provide damping for maximum frequency i.e. ${\omega }_n$ =2.65Hz =16.65 rad/sec

If the damping ratio (ρ) = $\frac{c}{c_c}=\frac{c}{2m{\omega }_n}=$ 1.0 then it is called critically damped system

Take Damping for 1^st, 2^nd, 3^rd storey $c_1=c_2=c_3$ = 1848150 N-s/m

Damping ratio $(\rho )=\frac{c}{c_c}=\frac{c}{2m{\omega }_n}=\frac{1848150}{2\times 55500\times 16.65}=\text{1}$

Hence it is critically damped

Take damping for 4^th storey $c_4=924075$

Damping ratio $(\rho )=\frac{c}{c_c}=\frac{c}{2m{\omega }_n}=\frac{924075}{2\times 27750\times 16.65}=1$

Hence it is critically damped.

Equivalent critically damped spring-mass system:

Equivalent critically damped spring-mass system for 4-storey building without any external force shown.

Transient Vibration Response graph for critically damped of Forced vibration System:

Initial time is 0.000001 sec.

Graph at time (t) = 0.001 sec

Graph at time (t) = 0.01 sec

Result for MDOF Critically Transient Response:

• Result for Max. & Min. Critically Transient Response of MDOF forced vibration of the damped system at two different time stages, i.e. (t)₁ = 0.001 sec, (t)₂ = 0.01 sec, with initial time 0.000001 sec presented in table 4.3

• Critically Maximum displacement overtime (t) = 0.01 sec presented in table 4.4

Over damped forced transient vibration response

Provide damping for maximum frequency i.e. ${\omega }_n$ =2.65Hz =16.65 rad/sec

If the damping ratio $(\rho )=\frac{c}{2m{\omega }_n}$ >1.0 then it is called overdamped system.

Take Damping for 1^st, 2^nd, 3^rd storey $c_1=c_2=c_3$ = 3696300 N-s/m

Damping ratio $(\rho )=\frac{c}{2m{\omega }_n}=\frac{3696300}{2\times 55500\times 16.65}$ = 2

Hence it is overdamped

Take damping for 4^th storey $c_4=1848150$ N-s/m

Damping ratio $(\rho )=\frac{c}{2m{\omega }_n}=\frac{1848150}{2\times 27750\times 16.65}$ = 2 Hence it is overdamped.

Equivalent Overdamped spring-mass system:

Transient Vibration Response graph for Overdamped of Forced vibration System:

The initial time is 0.000001 sec.

Overdamped forced vibration graph for time (t) = 0.001 sec

Overdamped forced vibration graph for time (t) = 0.01 sec

Result for MDOF Overdamped Transient Response:-

Result for Max. & Min. Overdamped Transient Response of MDOF forced vibration of the damped system at two different time stages, i.e. (t)₁ = 0.001 sec, (t)₂ = 0.01 sec, and initial time is 0.000001 sec, presented in table 4.5

Overdamped Maximum displacement over time (t) =0.01 sec presented in table 4.6

The Transient vibration response has done on ANSYS it gives sufficient results and graph between time and displacements also gives peak value of displacements for both undamped and damped systems of MDOF systems.

In the case of the damped system worked on all three conditions that are underdamped, critically damped and overdamped systems. According time-history graph and table can select the approximate damping value to the structures.

The time-displacement or deformation graph obtained for two different time stages. The natural frequency is achieved by both theoretical calculations and ANSYS. It is necessary for transient vibration response analysis is and it is easily determined by ANSYS. The whole study of transient vibration response makes possible to predict the damping values which resist any type of sudden impact or force vibration like blast, Earthquake and Tsunami etc. The ANSYS is the modelling and simulation software is used to perform the transient vibration response. The Mode Superposition method is used by ANSYS to calculate the structure response. The 4-DOF undamped & damped average value has shown below.

The 4-DOF undamped & damped maximum value has shown below.

The experiment shows that the value of damped transient displacement is less in comparison to the undamped transient displacement values. 4-DOF maximum undamped transient value is 0.020832 m and underdamped, critically and overdamped value is 0.019693m, 0.018678 and 0.016946 m. Therefore, this value shows that the damped transient displacement is less than the undamped transient displacement.